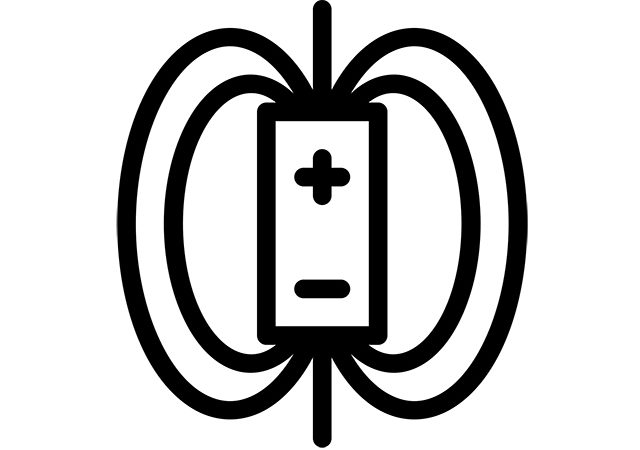
Let’s consider the solenoid that creates a magnetic field when DC current goes through the coil. The field in the solenoid is uniform, and it is proportional to the current through the coil:
If there is any substance in the solenoid, its magnetic field will change:
As soon as this field is characterised by a bigger intensity close to the nucleus, we have to use the average distributed magnetic field for calculations. To describe the magnetic field correctly, which is created by the micro-currents in the fragment of substance, we must consider the magnetising vector of substance:
The magnetising vector is a local characteristic, and can vary in different points of substance. This characteristic helps to determine the magnetic field:
For uniform and isotropic fields, magnetising is the following:
Where is a magnetic susceptibility coefficient. Thus,
The parameter shows how the magnetic field in the substance differs from magnetic fields in a vacuum –
is a magnetic permittivity.